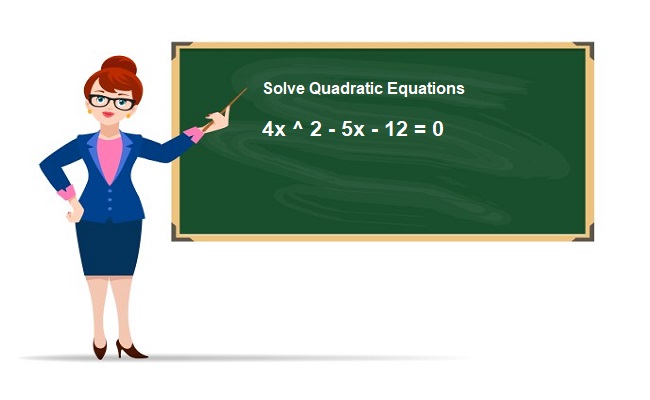
Algebra and quadratic equations never lack in a mathematics test. Learning to solve them is made easier with a step-by-step guide. Let us learn how to solve 4x ^ 2 - 5x - 12 = 0 in well-described steps in this article.
The goal is to find the value of x but we get to understand the variables and coefficients in the process. Our expression of interest above is a quadratic equation that is solved in easy steps. But first,
What are Quadratic Equations?
This is a mathematical expression best known as second-degree polynomial equations because they have some of the variables raised to the power of two. This complex quadratic equation is broken down by factoring in the general quadratic formula.
General Expression: ax2 + bx + c =0 where a, b, and c are the coefficients and x is the variable.
The coefficient must be a number between 1 and 9 since giving it a ‘zero’ value changes the equation form.
You will get the value of x by solving the quadratic equation
Characteristics of Quadratic Equations featuring 4x ^ 2 - 5x - 12 = 0
Here are some of the fundamental properties of this equation:
- It is parabolic in shape
- Has a vertex that shows the minimum and maximum points
Methods Applied in Solving Quadratic Equation 4x ^ 2 - 5x - 12 = 0
There are 4 different ways of solving this equation that lead you to the correct solution:
- Graphical Method
- Factorization
- Complete Square Method
- Using the Quadratic Formula
Let us solve it by:
Using the Quadratic Formula
This is achieved by applying the general quadratic formula. It is also easy to follow through and very reliable.
Follow these steps:
1: This works by substituting the values in the formula:
General Formula: x= -b ± √b2 – 4ac/2a
Equation to be solved: 4x ^ 2 - 5x - 12 = 0
Here are the coefficients: a=4, b=-5, c=1
2: On placing each coefficient in the right place, work it out as follows
X=-(-5) ± √ (-5)2 – 4(4)(-12) / 2(4)
X= (5) ± √(25) + (192) / 8
X= 5 ± 14.73/8
3: X has two values: Solve for each separately by applying + then -
x= 5+14.73/8 or x= 5-14.73/8
Which gives the value of x as:
X= 2. 467 and x= -1.216
You can evaluate and test your answer by substituting the value of x in the equation to prove that when x is solved, the net value of each side is zero.
Complete Square Method
Apply the following steps to solve for x
Reduce the coefficient of the first term to 1 by dividing the whole equation by 4
(4x ^ 2 - 5x - 12 = 0) /4
Results to:
X2- (5/4) x - 12 = 0
Next,3 is added both sides of the equation
X2- (5/4) = 3
To complete the square, divide the coefficient of x by 2 then square the answer
i.e. (5/4)/2 = 5/8 then square to get 25/64
Add the answer to both sides of the equation as follows:
X2- (5/4)x+ (25/64)=3 +25/64
This results to:
X2 – (5/4)x +(25/64) = 217/64
This completes the left-hand side to a perfect square as illustrated below
(x- (5/8)). (x-(5/8)) = (x-(5/8))2
Both sides are equal so they can do the same thing.
As per the law of transitivity,
(x-(5/8))2 = 217/6
Also, according to the Square Root Principle, their square roots are equal
Hence,
(x-(5/8)= √ (271/64)
Add 5/8 to both sides so that,
X= (5/8 ± √ (271/64)
This means x has two values
X= 2. 467 and x= -1.216
Using Factorization Method
In the factoring method, you need to discover two components: the binomials and clear all fractions.
These are the steps and how we can apply them to solve 4x ^ 2 - 5x - 12 = 0
Clearing the fractions in a case where they exist then write the equation in the general form of a quadratic equation:
ax2 + bx + c = 0
Our quadratic equation has no fraction segment hence step one does not apply
Factorizing by splitting the middle term
In 4x ^ 2 - 5x - 12 = 0
4x ^ 2 is the middle term with 4 as the coefficient
This makes -5x the middle term whose coefficient is -5
Our constant term is -12
Multiply the first term coefficient ‘4’ with the constant term ‘-12’
4x-12=-48
Figure out two factors of -48 that when added together equals the middle term
Example:
1 + -48= -47
-12+4 = -8
On trying for several, pick the pair that yields -5 when put together
No pair yields -5 so do not use factorization to solve this particular quadratic equation because it is trinomial.
This method best applies where the first term has ‘1’ as the coefficient.
Note: When using the quadratic formula, remember that a coefficient cannot be ‘zero’. This is because substituting zero to this equation converts it into a linear equation. Changing the form of the equation changes how it is solved too.
Conclusion
This article has outlined three methods and steps involved in the quadratic equation: 4x ^ 2 - 5x - 12 = 0. Among the three methods, using the quadratic formula has proved to be the easiest and most reliable. The Factorization method on the other hand failed in the last step since we could not get a matching pair of factors. Complete the square also works in solving this equation. Feel free to adopt the method that is simple to you. For more information visit techqiah.
